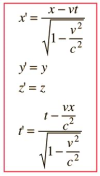Newtons laws, relativity & the ether

[woneill1701]

Here's a paper about the ether that someone posted on the Thunderbolts forum:



The Thunderbolts thread is here:



I have a few comments:

*I think the velocity vector field for the ether is the acceleration field for gravity.

*The guy who posted this on the Thunderbolts forum said that because fluid mechanics only works if the fluid can be treated as a continuum, then below a certain scale the theory doesn't work. This could mean that this theory is a classical theory and below a certain scale quantum theory is needed.

*I think the ether is relativistic so the velocity vector field will be v(x, t) for one observer and it'll be v(x', t') for another.

At risk of not being able to understand the maths, I am intrigued by your concerns with SR and the Lorentz transform.

Would you be interested in exploring that and bouncing some ideas around?

Obviously, I might not be up to being a realistic sounding board, but I am willing to try, and you know where I am coming from in terms of seeing SR as a potential answer to QM... (dimensions + time collapsing from the perspective of the wave...)

My math is is still very primitive (I’m currently studying basic proofs as a prerequisite to abstract algebra), but I can think oob and, if you are still forming theories, might be able to prompt questions and answers that might be able to trigger something profound...

 

[Guest 15258]

I borrowed new foundations for classical mechanics from my local library and I'm enjoying it. I don't think I'll read all of it though, it's a big book.

Would you be interested in exploring that and bouncing some ideas around?

Absolutely, I though no one would ever ask. :)

In SR there's the Twin paradox.

In physics, the twin paradox is a thought experiment in special relativity involving identical twins, one of whom makes a journey into space in a high-speed rocket and returns home to find that the twin who remained on Earth has aged more. This result appears puzzling because each twin sees the other twin as moving, and so, according to an incorrect[1][2] and naive[3][4] application of time dilation and the principle of relativity, each should paradoxically find the other to have aged less. However, this scenario can be resolved within the standard framework of special relativity: the travelling twin's trajectory involves two different inertial frames, one for the outbound journey and one for the inbound journey.[5] Another way of looking at it is by realising that the travelling twin is undergoing acceleration, which makes him a non-inertial observer. In both views there is no symmetry between the spacetime paths of the twins. Therefore, the twin paradox is not a paradox in the sense of a logical contradiction.

The above is the first paragraph on the Wikipedia page. The twin paradox is one of the reasons I think SR is wrong. I think the resolution to the paradox, whereby one observer is accelerating, isn't sound.

suppose we have two observers starting at the same position in completely flat space. At some time, say t = 0, they both start accelerating away from each other at the same rate, then they both decelerate at the same rate at the same time, turn around, accelerate towards each other at the same rate, decelerate again and finally come to rest again at the same point.

Somehow these observers must observe each other to have aged at the same rate, right?

 

[woneill1701]

I borrowed new foundations for classical mechanics from my local library and I'm enjoying it. I don't think I'll read all of it though, it's a big book.



Absolutely, I though no one would ever ask. :)

In SR there's the Twin paradox.



The above is the first paragraph on the Wikipedia page. The twin paradox is one of the reasons I think SR is wrong. I think the resolution to the paradox, whereby one observer is accelerating, isn't sound.

suppose we have two observers starting at the same position in completely flat space. At some time, say t = 0, they both start accelerating away from each other at the same rate, then they both decelerate at the same rate at the same time, turn around, accelerate towards each other at the same rate, decelerate again and finally come to rest again at the same point.

Somehow these observers must observe each other to have aged at the same rate, right?

Awesome!

This was the part about SR that, originally, totally griped me! (As opposed to gripped me!)

A regular perspective transform into the energy dimension would have restored both twins to the same age once they returned to the same origin.

It is like the concept of moving away from someone and appearing much smaller at a distance, and then moving back, and being restored to your original size! (Except, with Lorentzian relativity, their size is now permanently changed...j

We are now at the core of what separates Gallileian and Lorentzian relativity!

At its core, Galilean relativity is commutative and Lorentzian relativity isn’t!

(For those who are not familiar with the concept of commutativity, the concept can best be understood by thinking of rotation and translation: a translation by 5 in x, followed by a rotation by 90 degrees about the origin, is not the same as a rotation by 90 degrees about the origin followed by a translation by 5 in x... The idea is that two non-commutative operations in different sequence will result in a different final result.)

Where commutavity kicks in wrt Einsteinian relativity is the concept that one twin experiences aging more than the other!

This is the classic phenomenon of SR that all undergraduate Physicists are forced to accept, or risk expulsion from their course. (I was politely told by my lecturer not to attend any more of his lectures purely because I ASKED the question how/why this worked the way he described. When he responded with the statement, “but Einstein said,”, and I asked, “but why is it like that?” I was told not to come back...

There was no answer! The folks teaching the orthodoxy didn’t truly understand it!

Now, I don't say that it is all broken, and cannot determine which model is real (my maths is not good enough), meaning that I cannot guarantee that I can answer @Archaea’s question! But, we are now at the core of what separates “modern” relativistic physics from that of Newton and Galileo.

To me, this is the most fascinating/fundamental/wonderful part of physics and, if we can resolve this, it will solve most of Quantum Physics and push us into a model that will unify the fields...

Now, to “speak” the language of advanced Physics, we need to learn the Maths. (Imagine trying to describe concepts for which you don’t have the appropriate words...)

I am not a Mathematician. But, Maths is a language - like English, French, C, Java and Klingon...

The relationship between Maths and Language is that the language must be constrained such that its expressions relate to Reality. (Klingon is a language but it only has meaning within the Star Trek universe....)

The point being that a language can say anything, but it only means something when constrained by rules that represent reality.

This is the intersection between reality and the language of maths, and is the reason why maths is needed to understand physics...

The funnest part of this is that, to explain the non-commutativity aspect of relativity, we need to resort to the Minkowski model of spacetime - which I regard as false...

But, I’m happy to be proven wrong in the name of truth!

I’ll put together an analysis and we can shred it for the purpose of answering this most fundamental of questions! We will get to the truth!

Damn! I love this forum!

 

[woneill1701]

I borrowed new foundations for classical mechanics from my local library and I'm enjoying it. I don't think I'll read all of it though, it's a big book.

It is big, but, to me it was so profound that I devoured it! I loved the way it redefined mechanics using Pythagoras to prove the matrix structures related back to simple dimensional (Vector) constructs.

(That book is just the introduction to a whole series that relates all of modern physics back to a construct of Clifford algebras.)

It, for the first time, explained the relationship between vectors, matrices, and transforms.

Generalise that to Quaternions and Octonions and we are ready to truly understand how all this fits together!

I cannot think in terms of classic vectors any more. They are just a subset of a much more comprehensive entity.

 

[woneill1701]

I’ll put together an analysis and we can shred it for the purpose of answering this most fundamental of questions! We will get to the truth!

Damn! I love this forum!

So, for those folks not up on the whole Relativity thing, and the Lorentz transform, the background is this:

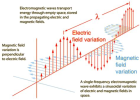
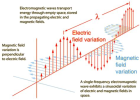
In the 1800s a scientist called Maxwell built upon the work of Faraday (and a number of other scientists of the day), to unify Electricity and Magnetism and come up with a set of wave equations that could be used to define the properties of Electromagnetic waves.

The work was total genius, and introduced the current conceptual model of the "EM" wave:



There was just one problem! Waves are understood to propagate through a medium, and the wave equations that define the wave motion always define motion relative to that medium.

Maxwell's equations didn't explicitly define the medium, but they did define something related: the speed of EM waves relative to that medium would always be constant - specified by the electrical and magnetic permittivity of the medium:

In this equation c is the speed, μ is the magnetic permittivity, and ε is the electric permittivity.

Both permittivities for free space had already been measured, and in empty space, c was predicted to be the speed of light we know today (186000 miles/second or 3e8 m/s).

This in itself was not startling to anybody, but a number of scientists of the day wanted to complete the theory by discovering the medium through which light was propagating. A number of experiments were attempted, but none were definitive until a US scientist called Michelson perfected the concept of an interferometer that would measure the "aether" as the expected medium was called:



Its way of detecting the effects of the aether was actually simple: measure the interference pattern generated by the two split beams of light, and then rotate the whole apparatus by 45%.

If there were any relative motion of this medium relative to the interferometer, then the interference pattern would change when the interferometer was rotated.

The reason was that if the speed of the light wave was fixed relative to the medium/aether and the interferometer was moving relative to the aether, then the paths to/from the two mirrors would take different times for each beam. By rotating the interferometer by 45%, you could offset the effects of any movement - changing the amount of time each beam took to bounce off the mirrors.

When Michelson and his friend Morley did the experiment, it failed. Not just once, but always.

Scientists all over the world then tried it, and Michelson spent the rest of his life repeatedly trying it. Even I did it in Physics Lab (and it didn't work for me either)!

Nobody was able to detect the aether or any movement through it. They tried different orientations, different rotations, but they never got a shift in the interference pattern.

An Irish scientist called Fitzgerald proposed that there must be some kind of compression/contraction happening as a result of moving through the aether, and two of the best scientific minds of the day, Poincare and Lorentz, took this idea and ran with it - developing what are today called the Lorentz Transforms:



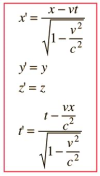
Originally, these transforms were meant to specify the compression of the measurement apparatus due to its movement through the aether.

Lorentz concluded that this compression was due to special behavior of a magical new particle that had just been discovered - the Electron... (Nobody really came up with a better explanation for years - even though the properties of the electrons were being investigated and didn't seem to be causing the contraction.)

It wasn't until a young Einstein proposed, over 10 years later, that there was no aether, and that the Lorentz transforms were universal, that things started to get surreal:

The consequence of Einstein's proposal was that without an aether/medium, light would always be measured as traveling at the same speed regardless of the relative motion of the light and any/all observer(s).

It also meant that the recently formulated vector-based mechanics that separated motion into components, aligned to a set of basis dimensions, did not apply to light: No matter which angle you measured it from, it would always be seen to travel at the speed of light.

Now, another implication - that even Einstein seemed to forget about - was that anything traveling at the speed of light would not experience either time or the universe through which it travelled - since due to the Lorentz equations, both time and space would be reduced to zero.

Most physicists will scoff that, "Einstein said that nothing can ever reach the speed of light" - without thinking about the fact that light can...

Actually if Lorentz holds, nothing with any mass can be accelerated up beyond the speed of light, because, its apparent mass would reach infinity, preventing any further acceleration. But light is the creature that caused the problem in the first place (and light is generally accepted to be massless)...

Now, shortly after Einstein re-interpreted the Lorentz transforms, a German scientist called Minkowski - a total mathematical genius - proposed a geometric way of representing some of the implications of the new Relativity.

This new geometric model was called Spacetime and essentially expanded the usual concept of graphing things against time to include our 3D world, time, and the effects of the Lorentz transform. This new 4D relativistic spacetime was invaluable for interpreting the implications of the new Relativity which were both mind-blowing and surreal - the most celebrated of them being the "Twin Paradox" referenced above by @Archaea which says that if one twin accelerated off in a spaceship and then came back, he would have aged less than the twin who stayed home and never experienced the same acceleration.

Not wishing to be outdone, Minkowski and his buddies than proposed that this 4D relativistic spacetime was not just a model, but was actually the reality in which we live, and that we are just moving too slowly to notice.

By doing-so, it introduced as "reality" the concept that time is actually another dimension.

After initially resisting, Einstein accepted this spacetime model and used it in General Relativity (which really just tries to unify all forms of acceleration - including spaceships and the result of gravity - into a coherent model).

I'll stop there with the history, but it leads in to my first question for @Archaea:

In your model, does the aether exist (and we have just not been able to detect it yet), or does light obey Einstein's theory that implies that it does not obey Newtonian/Galilean/Vector-based dynamics?

Or, do you have a model that transcends these concepts?

(For reference, Einstein's Relativity is still just a theory, and there are still many folks shooting at it!)

 

[woneill1701]

suppose we have two observers starting at the same position in completely flat space. At some time, say t = 0, they both start accelerating away from each other at the same rate, then they both decelerate at the same rate at the same time, turn around, accelerate towards each other at the same rate, decelerate again and finally come to rest again at the same point.

Somehow these observers must observe each other to have aged at the same rate, right?

Yup!

In SR the key is acceleration - if they both experience the same acceleration then they will both experience the same time.

The classic twin paradox results from only one twin experiencing acceleration (ignoring gravity...)

 

[Guest 15258]

It is like the concept of moving away from someone and appearing much smaller at a distance, and then moving back, and being restored to your original size! (Except, with Lorentzian relativity, their size is now permanently changed...j

I didn't think of it that way, I like that. I think that's a good way for us to think about it moving forward.

I think time dilation goes hand in hand with the Doppler effect. Suppose we have two observers in different inertial reference frames, and the first one has a clock. Now suppose that the first observer sends out a light pulse every time their clock ticks. The other observer will see the light pulses and the clock ticks line up in their inertial reference, since they are observing the first observers clock by way of light.

Now replace the light pulses with an EM wave and synchronize the peaks of the wave with the clock ticks, and you get time dilation corresponding with the Doppler effect. So if an observer is coming towards you, you observe their time going by faster, and if they receding, you observe their time moving slower. This resolves the twin paradox.

What do you think? Do you think this works?

This is the classic phenomenon of SR that all undergraduate Physicists are forced to accept, or risk expulsion from their course. (I was politely told by my lecturer not to attend any more of his lectures purely because I ASKED the question how/why this worked the way he described. When he responded with the statement, “but Einstein said,”, and I asked, “but why is it like that?” I was told not to come back...

Yeah, that's not right. I think it's a conspiracy. I think the conspiracy is maintained by people wishing to keep the correct ideas secret and the self-importance of people who don't know any better.

To me, this is the most fascinating/fundamental/wonderful part of physics and, if we can resolve this, it will solve most of Quantum Physics and push us into a model that will unify the fields...

I think if you just transform everything naively using the chain rule and keep the whole speed of light is constant in all inertial frames thing, there is no mismatch between QM and SR. The problems in mainstream physics are a result (at least partly) of SR being incorrect.

I think what we need is the link between EM and gravity. At the moment I quite like the idea that gravity is the Fourier transform of EM and exists in momentum space. But I dunno, I think doing the maths first step by step is good idea.

In your model, does the aether exist (and we have just not been able to detect it yet), or does light obey Einstein's theory that implies that it does not obey Newtonian/Galilean/Vector-based dynamics?

Or, do you have a model that transcends these concepts?

I don't have a model. The C's talk about the aether. There are some ideas in this thread that we can discuss later but I gtg now.

I like the constancy of light and principle of equivalence though.

Also, I hope you're not putting me on a pedestal, I'm not that smart and I'm not smarter than you. I'm keen on this stuff though, and I'm very interested in discussing.

Live long and prosper.

 

[woneill1701]

I think time dilation goes hand in hand with the Doppler effect. Suppose we have two observers in different inertial reference frames, and the first one has a clock. Now suppose that the first observer sends out a light pulse every time their clock ticks. The other observer will see the light pulses and the clock ticks line up in their inertial reference, since they are observing the first observers clock by way of light.

Now replace the light pulses with an EM wave and synchronize the peaks of the wave with the clock ticks, and you get time dilation corresponding with the Doppler effect. So if an observer is coming towards you, you observe their time going by faster, and if they receding, you observe their time moving slower. This resolves the twin paradox.

What do you think? Do you think this works?

The two are related but not the same.

The doppler effect affects the perceived clock/frequency by an observer in relative motion to the source. It works almost the same for sound as it does for EM. The crucial difference between doppler shift and time dilation is acceleration.

If two entities (with perfect clocks) are passing each other in opposite(ish) directions but NOT undergoing acceleration, then they will see each other’s clock running fast as they approach each other, then as they actually pass, they will see the other’s clock/frequency slow down and once they are moving away, they will see the other’s clock running slow.

Now, the important thing is that they do not experience any acceleration. Internally, they both age at the same rate.

This is equivalent to the scenario you proposed above where both experienced the same acceleration and both aged the same.

In the twin paradox, the twins undergo asymmetric acceleration (one accelerates, while the other does not).

This is where the Lorentz transform gets screwy...

While regular perspective is based on static distance, and commutes, the Lorentzian perspective transform is based on ”energy distance“ and does not commute. (I think this is what @JohnG meant when he associated time with a rotation on the other thread... Just guessing here...)

But the concept behind the Lorentz transform is that as the flying twin accelerates, they undergo a permanent asymmetric reduction in experienced time and distance.

Imagine it this way: if they screwed up and, instead of doing a round trip, managed to blast themself into the moon at 99% of the speed of light, they would experience a trip lasting only a few seconds before they went splat. They would have no time to change course.

The twin on the ground would see them take maybe 5 minutes before impact, and would not understand why they did not change course before impact...

This is why I always push the extreme by extrapolation to almost the speed of light (it is easier to visualize): Under Lorentz, the accelerated twin would experience almost zero time and see almost zero distance; meanwhile the un-accelerated twin would see finite speed, uncompressed distance, and uncompressed time.

 

[woneill1701]

Yeah, that's not right. I think it's a conspiracy. I think the conspiracy is maintained by people wishing to keep the correct ideas secret and the self-importance of people who don't know any better.

I definitely agree! My belief is that most of the folks - both teaching and learning - convince themselves that they understand it and that they are PHYSICISTS!

But none of them ever think about what they are either teaching, or being taught, and through this, the reality remains hidden!

I can understand it: if you were the CIA/NSA/MI6/KGB would you want some enthusiastic naiive young student telling the world about some new concept that would give the “enemy” the next new superweapon?

E=mc^2 unleashed atom bombs which are still being refined over 100 years later...

Anybody with the potential to develop such a new technology would need to be screened, groomed, and constrained such that they do not unleash a dragon!

 

[woneill1701]

I think if you just transform everything naively using the chain rule and keep the whole speed of light is constant in all inertial frames thing, there is no mismatch between QM and SR. The problems in mainstream physics are a result (at least partly) of SR being incorrect.

I think what we need is the link between EM and gravity. At the moment I quite like the idea that gravity is the Fourier transform of EM and exists in momentum space. But I dunno, I think doing the maths first step by step is good idea.

There IS a potential problem with this.

My maths is no-longer up to it, but from what I remember, while Lorentz was defining his transform, he ran everything through Poincare. Poincare was, in my mind, the true mathematical genius of the two. He ran the sanity checks on everything Lorentz came up with...

Basically, Poincare noticed some anomalies that resulted from the transform - long before Einstein or Minkowski got involved...

From what I can tell, it was Poincare that first identified the non-commutative relationship between time and acceleration, but he could not accept it, and backed-off. (Somebody correct me please!)

It was this non-commutative relationship that Minkowski used, years later, to justify his version of spacetime. He claimed that what Poincare had discovered was that Lorentzian acceleration corresponded to a rotation in hyperbolic Riemann spacetime... (Again, somebody correct me please!)

But, the end result was definitely that Minkowski and team rammed spacetime into the world as the new “reality”.

Overall, discounting Minkowski, the real issue is that Poincare realized that there were fundamental and profound implications associated with accepting the universality of Lorentz transforms. So much so, he clearly backed off from what would have been HIS relativity...

(To me, this is what happens when someone un-trained in “the work” looks into the abyss, and sees the abyss looking back! I see humanity pushed beyond its limits here - meaning truth...)

The issue comes back to whether Lorentz is universal, or whether there is an aether...

If Lorentz is universal then, to me, Poincare's reaction proves that acceleration really does cause a non-commutative change in experienced time. Yes, Minkowski hi-jacked it, and probably broke it, but Poincare‘s reaction tells me that it was this that Minkowski and team were trying to conceal...

I’m re-building my maths from scratch, but I’m no Poincare, and it will be a while before I can critique his (and Minkowsk’s) work.

So here, for now, I am operating at my limits...

But your maths is way beyond mine, and I believe we can still explore this aspect of reality and get to the truth...

Damn! I’ve never been so publicly exposed...

 

[woneill1701]

I think if you just transform everything naively using the chain rule and keep the whole speed of light is constant in all inertial frames thing, there is no mismatch between QM and SR. The problems in mainstream physics are a result (at least partly) of SR being incorrect.

I think what we need is the link between EM and gravity. At the moment I quite like the idea that gravity is the Fourier transform of EM and exists in momentum space. But I dunno, I think doing the maths first step by step is good idea.

I missed most of these vital points last night - they went over my head and I was focused on the Poincare thing and constant speed of light (it was nagging at me...)

You make a number of points that are intriguing:

I think if you just transform everything naively using the chain rule and keep the whole speed of light is constant in all inertial frames

How would this chain rule be applied? Are we looking at Galileian transforms or Lorentzian Transforms being chained, or something different?

Keeping the speed of light constant and applying Lorentz has implications (as Poincare found). I'll see if I can find some "light reading" about his and Lorentz's original maths...

To me, if we do keep light constant and apply Lorentz, then it answers so many of the apparent insanities associated with the SR/QM thing - even though Einstein and Bohr liked to argue over it... (Though thinking deeper, it might have been Heisenberg's uncertainty model that Einstein was objecting to. That said, it still doesn't explain Einstein's concern about "spooky action at a distance" if, by his own (Lorentz's) maths, said distance doesn't exist...)

I think what we need is the link between EM and gravity. At the moment I quite like the idea that gravity is the Fourier transform of EM and exists in momentum space. But I dunno, I think doing the maths first step by step is good idea.

To me, if we accept the invariance of c (the speed of light is typically referred to as "c" in physics books), then charge and mass are equivalent things translated/rotated and expressed through different dimensions:

• Mass exists as a fundamental "thing" in our 3D universe and either generates/results-from a gravitational field
  • Movements of mass generate waves that have strange "QM" properties that cannot directly be detected in our 3d universe, and in many ways seem to display properties that are both time related and time independent - hence the "statistical" aspects of QM including wave-particle duality
• Charge exists as a (fundamental?) thing in our 3D universe and either generates/results-from an electric field
  • Accelerations of charge generate waves that have strange QM/Relativistic properties that can directly be detected in our 3D universe, but themselves do not experience our universe, and in many ways seem to display properties that are both time related and time independent - hence wave-particle duality

To me, the symmetries are obvious:

If the electric field is a property of charge, and acceleration of charge generates E/M waves, and if the Gravitational field is a property of mass, and movement of mass generates Quantum waves then these Quantum Waves are really the elusive Gravitational Waves that "scientists" are struggling so hard to find!

To me, they are all related through particles that have properties that overlap dimensions:

• Mass has three dimensional "physical existence" and generates gravitational ripples in one or more additional dimensions when it moves; we don't see those other dimensions because we are bound by time, which, as we know it, only exists in our three.
• Charge has properties in our three dimensions and generates E/M ripples when it is accelerated. But the E/M ripples have no experience of time, and two components: Electricity that expresses itself in one dimension, and Magnetism that is the same expression of Electricity through a second dimension....

For me, I see a particle interacting with maybe 5 dimensions of a 16D universe. Some particles overlap which dimensions with which they interact. We are physically aware of only 3 because our brains are physically limited such that we can only measure things for which time exists as a consequence of movement. (We are incapable of directly detecting things that don't experience time!)

But with the right combination of Geometry and algebraic rules, we can do what all science is supposed to do: make testable predictions!

One day my maths will be up to deriving this mathematically, but for now it must wait...

At the moment I quite like the idea that gravity is the Fourier transform of EM and exists in momentum space.

Interesting! Fourier analysis really just identifies frequency components of a fluctuating value over time. I'm big into DSP so understand Fourier quite well...

I'm intrigued as to how would that produce gravity.

I get how a wave packet can be decomposed into frequency space, and understand how momentum appears to be a highly significant player in theoretical physics (light has no mass, but does have momentum etc., Heisenberg's uncertainty is expressed in terms of momentum, and De Broglie's wave equation is also expressed in terms of momentum.)

In QM, momentum does appear to have profound significance beyond its Newtonian interpretation. I never really questioned that significance... Time to do that!

Maybe Momentum in our Mass-oriented 3D directly relates to Energy in a Charge-oriented universe...

In Newtonian calculus, Energy is just an integral of Momentum wrt velocity: p=mv vs E=1/2 mv^2...

But, how would Fourier be applied?

I get the relationship between momentum and frequency:



And the relationship between mass and Gravity ==> meaning that Momentum (typically labeled "p" in most Physics books) - even for massless particles - can potentially generate a Gravitational field... (I'm trying to remember if I ever encountered the concept of gravitational attraction between two photons. There does seem to be some vague recollection in there, but I'm getting nothing concrete...)

But I don't see how you would get there via Fourier analysis.

I suppose we are back to my limited maths beyond the world of DSP...

Back to doing my impression of a chimp with an old twig...

Apologies for not being able to fully explore your hypothesis!

But I dunno, I think doing the maths first step by step is good idea.

Did you ever use Minkowski spacetime diagrams to explore the geometry of SR? (They were constructed as a geometric expression of the maths of Lorentz/Poincare, and as far as I can tell, correctly reflect the implications of the "Lorentz Transform" - if it is universally true...)

I still don't accept the Minkowski theory that his Spacetime is real, but his diagrams are one of the most elegant constructs ever in enabling an understanding of the geometric implications of SR...

 

[woneill1701]

Keeping the speed of light constant and applying Lorentz has implications (as Poincare found). I'll see if I can find some "light reading" about his and Lorentz's original maths...

Wow!!! Have I got something for you!!!

Link to free article published by Cornell University that gives a really deep treatment of the maths developed by Poincare that formed the foundation of SR...

 

[Guest 15258]

If two entities (with perfect clocks) are passing each other in opposite(ish) directions but NOT undergoing acceleration, then they will see each other’s clock running fast as they approach each other, then as they actually pass, they will see the other’s clock/frequency slow down and once they are moving away, they will see the other’s clock running slow.

Now, the important thing is that they do not experience any acceleration. Internally, they both age at the same rate.

Ok, I'm very happy... I think we're on the same page regarding this.

Wrt the Lorentz stuff, are you happy to treat it as not needed for the moment, or do you want to work through it? Here are two of my old threads,

An alternative derivation of special relativity
Alternative quantum theory

Most of the SR one is garbage, but in post #52 Ronan points out some video where SR is derived. The interesting thing is that the constancy of light is never used as far as I recall. The QM thread still has some things I like I think, I haven't looked at these threads in a while.

I can understand it: if you were the CIA/NSA/MI6/KGB would you want some enthusiastic naiive young student telling the world about some new concept that would give the “enemy” the next new superweapon?

E=mc^2 unleashed atom bombs which are still being refined over 100 years later...

Anybody with the potential to develop such a new technology would need to be screened, groomed, and constrained such that they do not unleash a dragon!

Right, but I think this might be an effect of false and limiting beliefs.

How would this chain rule be applied? Are we looking at Galileian transforms or Lorentzian Transforms being chained, or something different?

I was thinking about this this morning. If we have a "spacetime block" then we need to use the multidimensional chain rule. But if we have time as a parameter and somehow have dx/dx' = dt/dt' then you can do things like P' = ih d/dx' = ih (dx/dx') d/dx = (dx/dx') P. But take this with a grain of salt. I feel like if we get on the same page regarding time and space dilation. we can start the maths stuff from scratch.

To me, if we accept the invariance of c (the speed of light is typically referred to as "c" in physics books), then charge and mass are equivalent things translated/rotated and expressed through different dimensions:

• Mass exists as a fundamental "thing" in our 3D universe and either generates/results-from a gravitational field
  • Movements of mass generate waves that have strange "QM" properties that cannot directly be detected in our 3d universe, and in many ways seem to display properties that are both time related and time independent - hence the "statistical" aspects of QM including wave-particle duality
• Charge exists as a (fundamental?) thing in our 3D universe and either generates/results-from an electric field
  • Accelerations of charge generate waves that have strange QM/Relativistic properties that can directly be detected in our 3D universe, but themselves do not experience our universe, and in many ways seem to display properties that are both time related and time independent - hence wave-particle duality

I don't know. I think mass might be like charge in momentum space or something...

But, how would Fourier be applied?

In my head I was thinking that you could take the Fourier transforms of Maxwell's equations, and this would give you quantities in momentum space that correspond to the electric and magnetic fields. Then I thought that maybe these quantities could be related to gravity.

Basically the idea was that there might be analogues of Maxwell's equations in momentum space, or that Maxwell's equations work in momentum space as well as position space, and that the momentum versions relate to gravity.

This idea doesn't seem quite so sound now that I'm writing about it though.

Have you done any real analysis or differential geometry?

I don't think we'll need this stuff, but I ask anyway. I gather that you calculus and vector calculus, since you know geometric algebra.

I think at this stage all we need is geometric algebra.

 

[woneill1701]

Wrt the Lorentz stuff, are you happy to treat it as not needed for the moment, or do you want to work through it?

I'm good if you are! My take is that we can use the exercise to explore any areas where either the PTB of the day may have intervened to limit/corrupt any further research down a given path, or the scientists of the day may have overlooked something useful (hindsight is 20:20 as they say...).

The last month for me has been fascinating because I started researching into the history behind the way the decisions/discoveries were originally formed, and how they were just as much the result of politics as they were science! Last night I was reading about the war between Gibbs/Heaviside and Hamilton/Tait over the question of whether to base the emerging Physics on Vectors or Quaternions... Even the top minds of the day made some atrocious decisions driven by expediency, animosity, and ambition.

Fascinating stuff!

Here are two of my old threads,

An alternative derivation of special relativity
Alternative quantum theory

Working through them now. May take me a while...

Right, but I think this might be an effect of false and limiting beliefs.

Yup! Add a dash of "fear of the unknown" to the politics and personalities of the day, and it is a wonder that anything ever got discovered.

I was thinking about this this morning. If we have a "spacetime block" then we need to use the multidimensional chain rule. But if we have time as a parameter and somehow have dx/dx' = dt/dt' then you can do things like P' = ih d/dx' = ih (dx/dx') d/dx = (dx/dx') P. But take this with a grain of salt. I feel like if we get on the same page regarding time and space dilation. we can start the maths stuff from scratch.

Sounds intriguing - but beyond my maths today! The time dilation stuff is definitely the key to most of it, but we also need to add Heisenberg and his uncertainty principle too! It could be just a property of wave functions, or it could be the most profound thing of all! I don't know yet... (I still have to research how/why Heisenberg got to his theory using Planck's constant...)

I think mass might be like charge in momentum space or something...

I do think that mass and charge will probably resolve to be the same thing experienced through a different space. Figuring out that is my long term aim...

In my head I was thinking that you could take the Fourier transforms of Maxwell's equations, and this would give you quantities in momentum space that correspond to the electric and magnetic fields. Then I thought that maybe these quantities could be related to gravity.

Did you know that Maxwell's Equations are not?

What we call Maxwell's Equations were re-formulated (and massively simplified/condensed) by Heaviside to use his new formuation of Vectors. His "Maxwell's Equations" look nothing like the original ones.

At some point I will re-explore Maxwell's original equations through the lens of Geometric Algebra and see if I get the same results as Heaviside, or whether there was something he missed... (Heaviside was an Electrical Engineer and wanted an E/M model that he could use as the basis of his new Transmission Line theory. I wouldn't be surprised if there were things in the original Maxwell Equations that he overlooked or dismissed as superfluous...)

But, beyond Maxwell: His equations were purely classical and predated the concept of a photon as a wave packet.

To bridge the gap between E/M and QM, you would also need to bring in Heisenberg and Schroedinger...

In this case, I am not mentioning Heisenberg in terms of his uncertainty principle, but in terms of his algebraic description of quantum waves: Both Heisenberg and Schroedinger came up with mathematical representations of "Quantum" wave packets at the same time. Schroedinger's was formulaic and could be understood/manipulated by the physicists of the day; Heisenberg's was based on Matrix operations...

But, they both gave the same results to the same situations, and Schroedinger's formulation was "chosen" because it was easier to re-state it in terms of the new Vector maths from Heaviside and Gibbs...

Have you done any real analysis or differential geometry?

I don't think we'll need this stuff, but I ask anyway. I gather that you calculus and vector calculus, since you know geometric algebra.

I never did any Real analysis. That is to come as part of my roadmap...

My original knowledge of Vectors and differential geometry was physics-based, process-oriented and limited. They teach you only enough of the maths to be able to do certain things with it. Additionally, mine has atrophied for 30 years.

This is part of my maths roadmap: to re-learn everything (including GA) from scratch through a linguistic/mathematical lens based on a universal number system and algebra, and with a fundamental understanding of why things are the way they are - not just, "this is a hammer: use it to knock in nails..."

One of the fundamental issues of the day that constrained the original scientists was that the number systems of the day were incomplete.

Hamilton and Gauss were exploring the geometry of complex numbers as an extension to Reals. Hamilton tried both to extend this geometry to represent the Real world (pun intended), and to extend the number system beyond the "2D" complex plane to expand it up to 3D and 4D and Beyond...

Meanwhile Gibbs and Heaviside wanted a geometric formulation that was more "Real" (again, pun intended), and formed, between them, the Vector model - deliberately excluding any complex/imaginary terms, and obscuring the relationship between numbers and geometry.

But, both models were incomplete...

Grassmann came up with a third model that merged the two, but being a lowly school teacher, was universally ignored, and Grassmann eventually dropped it and became a linguist. Many years later this was recognized by Clifford as a more universal number/algebraic system that could unify geometry and algebra. But when Clifford died, nobody ran with it until Hestenes began publishing it as the answer to life, the universe, and everything...

I think at this stage all we need is geometric algebra.

It is definitely a start. For you, it may be enough to complement your existing mathematical understanding and round things out.

For me it is only the first rung on the ladder of understanding a unified mathematical model that includes numbers, geometry and algebra.

I have to start again from scratch and re-learn calculus from the dual perspective of a mathematician who knows GA. Then apply it to multidimensional Geometry, Maxwell and Heisenberg/Schroedinger...

With the maths we would need to be able to generalize wave packet formulations across relativistic boundaries: I've never yet seen a unification of the classical E/M wave model with that of a photon wave packet.


vs:



And it's not as simple as applying a gaussian envelope to the Maxwell wave: The Maxwell wave is a physical wave that has polarization and measurable properties over time that can be both generated and detected in an antenna, while the Quantum wave packet is a purely statistical probability model. (Despite the obvious visual and structural symmetry...)

Now, by eliminating their experience of time, we can make photons also a purely statistical thing as well. But then how do we generate them with an antenna?

To get there we would need to account for an explanation of how a photon can be polarized in our universe if our universe doesn't exist to it. What does polarization mean to light? Does it even exist to it?

Quantum waves have something called spin - that behaves similarly to polarization. Is it the same thing in another space? Or, is there something different that also needs to be accommodated?

Similarly, we would need to unify field theory, classical antenna theory - dynamic fields and the generation of polarized E/M waves via the oscillatory linear movement of charge in a wire - with the photonic/quantum model whereby an arbitrarily polarized photon is generated from a change in the energy level/state of a charged particle...

(Laser physics - which is real and exists - is based on the quantum photon model, and relies on the "stimulated" emitted photon being coherent in phase/frequency and polarization with the "stimulating" photon... Again, I've never seen a good explanation as to why it works - only that it does...)

This would all need to be reconciled with the concept of E=mc^2 and an electrical/charge counterpart...

And at this point, we haven't even started to answer the question: But why do we experience time when the wave packet doesn't...

Where I am going with this is that we need the maths to be able to unify Lorentz/time dilation with wave-packets that don't experience a "space" but are clearly experienced within that space, interact "classically" in that space, and which have clearly measurable properties within that space.

What kills me today (and did-so 30 years ago) is that I can visualize all these interactions, but do not have a mathematical language that can express them, and explain the origins of the rules that govern them.

 

[woneill1701]

Ok, I'm very happy... I think we're on the same page regarding this.

Wrt the Lorentz stuff, are you happy to treat it as not needed for the moment, or do you want to work through it? Here are two of my old threads,

An alternative derivation of special relativity
Alternative quantum theory

Most of the SR one is garbage, but in post #52 Ronan points out some video where SR is derived. The interesting thing is that the constancy of light is never used as far as I recall. The QM thread still has some things I like I think, I haven't looked at these threads in a while.

I just watched the two videos where SR is derived. They are wrong! Not just a little bit, but completely!

The professor is obviously very clever, and his maths are correct and elegant, as far as they go. But he should have spent more time researching SR.

What he was deriving was equivalent to Galileian Relativity using AEtheric light as a clock. Had Michelson-Morley worked, his spacetime model would have been correct.

In the second video his treatment of the experience of B uses Galileian calculations for x' and t', which would only hold if the waves were passing through a fixed aether. (In his videos, the speed of the clock pulse is always calculated from the reference frame of an observer at rest wrt the medium and watching both A and B).

I was surprised that my maths was still able to catch him...