Erdos idea
A Disturbance in the Force
Thank you MJF, Bluegazer (and Laura and Ark) and others. . .
I offer my pennies in the till:
I had an interesting idea today from the BBC In Our Time podcast. The topic was Paul Erdos. Went into Erdos' work in graph theory and Ramsey theory. What struck me is Erdos was at the Institute for Advanced Studies in 1938ish? His fellowship was not renewed supposedly because he was "uncouth" but Einstein was unhappy with this and tried to keep him. After this Erdos was itinerant, always meeting other mathematicians and working collaboratively on problems, including graph and Ramsey theory, eventually being banned from returning to the U.S. due to potential communist sympathies (though I smell a cover story).
My thought was what might Einstein have told him during his time at Princeton? Perhaps a clue as to the unified field Einstein was silenced to speak about? Could it relate to the graph theory / Ramsey theory which was such a focus for Erdos in his wandering years? Was he trying to work out independently/encourage others to work out what was silenced by the COINTELPRO forces? I am only speculating, but made the connection this morning while listening to the podcast. Your comments about Ramsey theory reminded me of the connection I made this morning and I made this account to share it.
Secondary thought is if Ramsey theory relates to "minimum conditions for order" could there be a connection between this idea and an FRV notion of graduated "levels" (I hesitate to say "densities" without more research) based on prime numbers, where each prime number represents a unique (because prime) "level" of order or organization. A sort of infinitely expanding series of "realms" (possibly, just using that term as a springboard). Would also explain the feeling of "compression" accompanying an attempt to force a "higher-prime FRV" into a more constrained "lower-prime FRV". Although I say infinitely expanding, my further thought is that in some way using p-adic moduli would mean that "infinitely" still relates or circles back, so to speak, maybe as a function of powers? Very vague, I know, but I wanted to share the idea, even if it is word salad.
An important point would be the idea that the larger the prime, the more "degrees of freedom" in each prime number-based FRV/Realm and/or the fewer "laws" (in the Gurdjieffian sense). Fewer laws means "higher" density/more degrees of freedom.
Perhaps Erdos was trying to work this idea out through graph theory/Ramsey theory from a hint or two from Einstein? And a knowledge of the political realities. Only speculation of course, these ideas sprang from the podcast today.
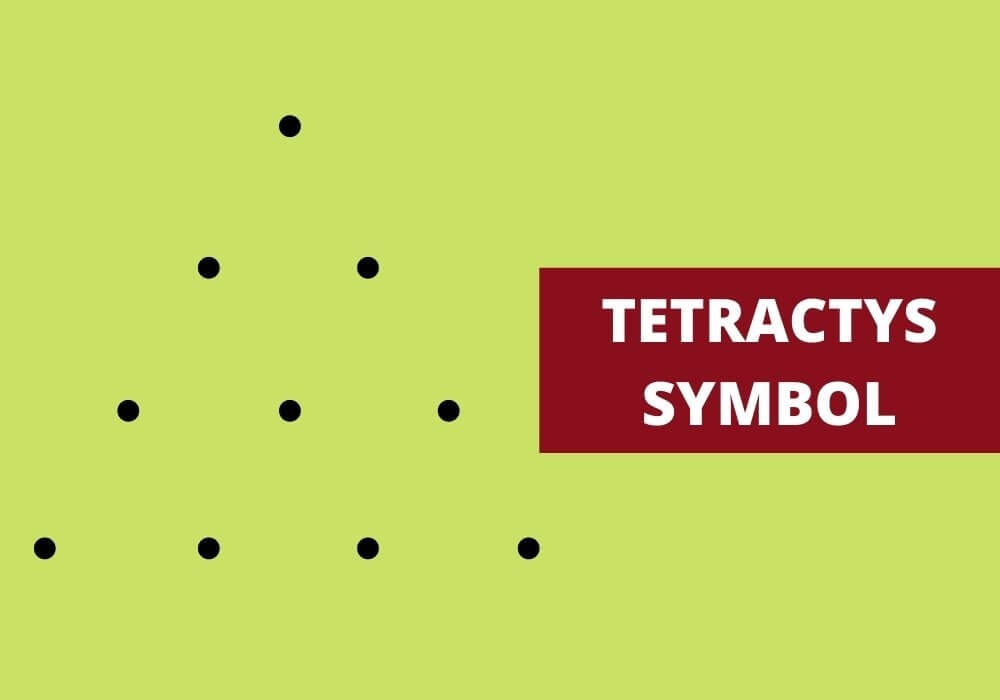
Finally, the question of the "prime gap". Perhaps there is a way to model this pattern (or discern it), the difference between the primes, but modeled in a 3d pyramid shape? As suggested in one of the sessions from 1997-98, if I recall correctly, rather than in a simple "n" type formula often used to define for example the natural numbers (I'm thinking of n(n+1)/2 type definitions). The formula would have to rely on quaternions or octonions or whichever is needed, to account for the 3d nature of the pyramidal distribution of the primes (or the flattened pyramidal distribution? Could this "flatness" relate to the first few primes, i.e. 1, 2, 3? Could 1, 2, 3 be the capstone, the cornerstone that the builders rejected?).
Once again I apologize if this is word salad or irrelevant to the question of what Francis Bacon was up to, but I wanted to share the idea I had, hopefully to the benefit of the network, and I created this account for that purpose. I now retreat back from whence I came.
Ave Caesar, and blest wishes for the network.
I offer my pennies in the till:
I had an interesting idea today from the BBC In Our Time podcast. The topic was Paul Erdos. Went into Erdos' work in graph theory and Ramsey theory. What struck me is Erdos was at the Institute for Advanced Studies in 1938ish? His fellowship was not renewed supposedly because he was "uncouth" but Einstein was unhappy with this and tried to keep him. After this Erdos was itinerant, always meeting other mathematicians and working collaboratively on problems, including graph and Ramsey theory, eventually being banned from returning to the U.S. due to potential communist sympathies (though I smell a cover story).
My thought was what might Einstein have told him during his time at Princeton? Perhaps a clue as to the unified field Einstein was silenced to speak about? Could it relate to the graph theory / Ramsey theory which was such a focus for Erdos in his wandering years? Was he trying to work out independently/encourage others to work out what was silenced by the COINTELPRO forces? I am only speculating, but made the connection this morning while listening to the podcast. Your comments about Ramsey theory reminded me of the connection I made this morning and I made this account to share it.
Secondary thought is if Ramsey theory relates to "minimum conditions for order" could there be a connection between this idea and an FRV notion of graduated "levels" (I hesitate to say "densities" without more research) based on prime numbers, where each prime number represents a unique (because prime) "level" of order or organization. A sort of infinitely expanding series of "realms" (possibly, just using that term as a springboard). Would also explain the feeling of "compression" accompanying an attempt to force a "higher-prime FRV" into a more constrained "lower-prime FRV". Although I say infinitely expanding, my further thought is that in some way using p-adic moduli would mean that "infinitely" still relates or circles back, so to speak, maybe as a function of powers? Very vague, I know, but I wanted to share the idea, even if it is word salad.
An important point would be the idea that the larger the prime, the more "degrees of freedom" in each prime number-based FRV/Realm and/or the fewer "laws" (in the Gurdjieffian sense). Fewer laws means "higher" density/more degrees of freedom.
Perhaps Erdos was trying to work this idea out through graph theory/Ramsey theory from a hint or two from Einstein? And a knowledge of the political realities. Only speculation of course, these ideas sprang from the podcast today.
Finally, the question of the "prime gap". Perhaps there is a way to model this pattern (or discern it), the difference between the primes, but modeled in a 3d pyramid shape? As suggested in one of the sessions from 1997-98, if I recall correctly, rather than in a simple "n" type formula often used to define for example the natural numbers (I'm thinking of n(n+1)/2 type definitions). The formula would have to rely on quaternions or octonions or whichever is needed, to account for the 3d nature of the pyramidal distribution of the primes (or the flattened pyramidal distribution? Could this "flatness" relate to the first few primes, i.e. 1, 2, 3? Could 1, 2, 3 be the capstone, the cornerstone that the builders rejected?).
Once again I apologize if this is word salad or irrelevant to the question of what Francis Bacon was up to, but I wanted to share the idea I had, hopefully to the benefit of the network, and I created this account for that purpose. I now retreat back from whence I came.
Ave Caesar, and blest wishes for the network.