Introduction:
All magnets found in nature are dipoles, having both a north and south pole. If a bar magnet is broken into two pieces, each piece become a dipole. It has been speculated, that by a natural extension of Maxwell's equations magnetic monopoles might be possible. A monopole would only be a north or south pole, with no accompanying opposite pole, not unlike a normal electric charge.
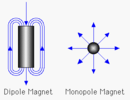
There have been some interest in the possibility of monopoles, mainly for theoretical purposes. In [2], my erstwhile colleague Daedalus sketched one method of constructing a monopole by assembling it from pyramidal bar magnets. This has some drawbacks (like the impossibility to reverse the polarity if needed), and was subsequently ridiculed by established science in [3] and [4].
Construction:
My own construction is based on electromagnetic effects and does not need any permanent magnets to work. We begin with placing six electrical coils at right angles to each other, along the standard co-ordinate axes. When current is led through them, a magnetic field results which will (if the polarity is right) enter through the coils and escape the core through the interstices between the coils. However, if the coils are made so that their cross sections are square, they can be moved together to form a cubical interior completely surrounded by coils with no place to escape. When current is applied, the magnetic field will only be able to enter the interior, not escape, and we have a magnetic monopole.

The main problem with this arrangement is that it is cumbersome and requires a constant supply of current. However, it can be elegantly generalised: imagine that each of the coils is pressed together into a square, so that we have a cube whose edges consist of coils, in which current flows.
Along each edge, the current on one side flows in one direction and on the other side in the opposite direction. This obviously cancels out both currents, which shows that a monopole does not require any external current, just like a magnetic dipole doesn't. The only thing which is needed is a closed conductive surface, so that the virtual vortices of virtual current can produce a real magnetic field.
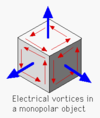
Why isn't normal conductive objects monopoles? The explanation is simple; since their fields are created by virtual electric currents, they can be directed in both the inward and outward directions, and without any outside influence both fields cancel each other. But if the fields are pushed in one direction, the object will become a monopole. This requires that the fields are strong enough to overcome the magnetic momentum of the object, which depends mainly on the material, its size and symmetry. Symmetric objects become monopoles much easier than asymmetric objects.
To push the fields in the right direction, we need a radial magnetic field. This is most easily created by another monopole placed around (or inside) the object. The above mentioned cubical arrangement of coils works fine for this. In my experiments I have used coils with a side of one meter, linked together by an supporting aluminium framework to build a monopolar charging unit. When a sufficiently strong current is applied, the monopolar fields force the virtual vortices of the object placed in the interior chamber to align and the object becomes a permanent monopole.
The most important variable in this process is the strength of the field, which is proportional to the current. One way to improve the efficiency is to use a single strong pulse instead of a constant current. One arrangement I have used is a bank of capacitators, whose energy is released by short-circuiting them. This places great demands on the cooling and structural stability of the system, which limits their use somewhat (superconducting coils would ameliorate the heat problem a great deal). Care must also to be taken so that the fields are exactly radial, since if the alignment of the coils is not correct, a powerful electromagnetic vortex will result which will destroy the object and possibly damage the coils. Current pulses are safer in this respect, but has an unfortunate tendency to cause electric discharges between different parts of the system if not properly insulated.








