Inspired by the recent discussions about crop circles, I decided to model reality using only circles... and a few arrows!
Let's see how deep the rabbit hole goes!
Introduction
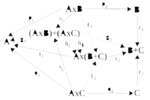
Since "consciousness is matter and matter is consciousness," every circle corresponds to a consciousness (unit).
Arrows represent directional movement, rotations, or state transitions.
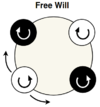
Free Will
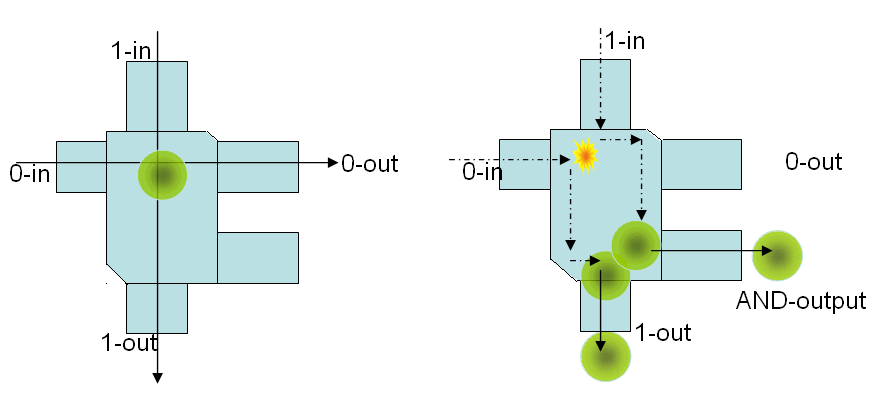
Each circle can freely spin (clockwise or counter-clockwise) around itself or move along the edge of the biggest circle, i.e. 7th density. Circles can also collide with each other. Guess which ones don't impose their will on others.
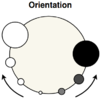
STO/STS Orientation
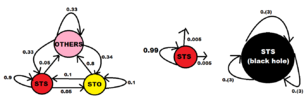
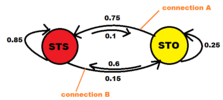
Each consciousness unit is free to chose whether it wants to feed on positive (light, objective) or negative (dark, subjective) energy. Consciousness units are initially "objective until they choose to be subjective." The size of each circle corresponds to the number of lessons learned. Bigger circles have greater awareness and knowledge.
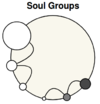
Soul Groups
A circle can be a member of a soul group which is "an affiliation that can be due to origin or graduation."
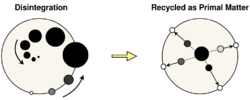
Disintegration
Once a circle grows past the threshold of negativity (4D STS), it starts to decay. It is absorbed by a black hole. The cycle is complete when primal matter, as a result of the disintegration, is given a new chance to learn, and to be in "union with the One."
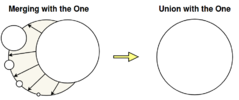
Union with the One
Circles on the STO path are not subject to disintegration. Once they "learn all their lessons," they effectively become "One," i.e. the system, the structure that they once sought to master.
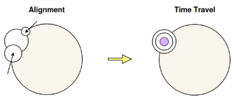
Time Travel
Circles align with "themselves in the future" or "themselves in the past" to access knowledge that is not known to them.
Conclusion
As you can see, circles are very versatile shapes! There are only so many "degrees of freedom" to explain the unexplained from a two-dimensional perspective. However, surprisingly, I think there is some logical foundation to this visual exercise, possibly due to the inherent properties of circles. Shapes seem to have "universal truths" embedded in them. Plus, the act of organizing shapes gives them additional meaning. Is there such a concept as "geometric logic?"
Let's see how deep the rabbit hole goes!
Introduction
Since "consciousness is matter and matter is consciousness," every circle corresponds to a consciousness (unit).
Arrows represent directional movement, rotations, or state transitions.
Free Will
Each circle can freely spin (clockwise or counter-clockwise) around itself or move along the edge of the biggest circle, i.e. 7th density. Circles can also collide with each other. Guess which ones don't impose their will on others.

STO/STS Orientation
Each consciousness unit is free to chose whether it wants to feed on positive (light, objective) or negative (dark, subjective) energy. Consciousness units are initially "objective until they choose to be subjective." The size of each circle corresponds to the number of lessons learned. Bigger circles have greater awareness and knowledge.

Soul Groups
A circle can be a member of a soul group which is "an affiliation that can be due to origin or graduation."

Disintegration
Once a circle grows past the threshold of negativity (4D STS), it starts to decay. It is absorbed by a black hole. The cycle is complete when primal matter, as a result of the disintegration, is given a new chance to learn, and to be in "union with the One."

Union with the One
Circles on the STO path are not subject to disintegration. Once they "learn all their lessons," they effectively become "One," i.e. the system, the structure that they once sought to master.

Time Travel
Circles align with "themselves in the future" or "themselves in the past" to access knowledge that is not known to them.

Conclusion
As you can see, circles are very versatile shapes! There are only so many "degrees of freedom" to explain the unexplained from a two-dimensional perspective. However, surprisingly, I think there is some logical foundation to this visual exercise, possibly due to the inherent properties of circles. Shapes seem to have "universal truths" embedded in them. Plus, the act of organizing shapes gives them additional meaning. Is there such a concept as "geometric logic?"
Q: Wait, I asked what is the second loop. The second loop is included but not inclusive?
A: Remember, you do have cycles but that does not necessarily mean cyclical. 3 Dimensional depiction of loop, seek hexagon for more. Geometric theory provides answers for key. Look to stellar windows. Octagon, hexagon, pentagon.
Q: Are those the different levels of density?
A: No, but it relates. Geometry gets you there, algebra sets you "free."