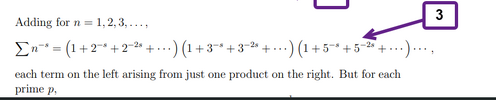Some comments on information theory

  • Thread starter Thread starter Cleopatre VII
  • Start date Start date
Natus Videre said:
Compressing Information
It would be quite fascinating if we could construct a special mathematical function which, when evaluated repeatedly, would produce a message of our choice.

fx.png
Click to expand...
This is more or less how our vocal communication occurs. We code certain information with sound waves and other person decodes these waves into the same (in most cases :D) information in same language.
 
PabloAngello said:
This is more or less how our vocal communication occurs. We code certain information with sound waves and other person decodes these waves into the same (in most cases :D) information in same language.
Click to expand...
During vocal communication, the whole message is sent in separate chunks, which is not exactly what I was aiming for.
Laura said:
Q: (L) Is there some way to communicate with whales or dolphins and can one find a way to translate the differences and have a reasonable, intelligent exchange with a whale or a dolphin or even an elephant?
A: You don't need conversation "with" when a higher telepathic level.
Q: (L) Dolphins and whales communicate telepathically?
A: Yes. So do dogs and cats and snakes etc. etc. only humans have learned the "superior" art of verbal communication.
Q: (L) But, at the same time, verbal communication can be quite limiting, is that correct?
A: That is the point.
Click to expand...
In order to communicate, we imprint our thoughts in a physical medium (like paper, air, electric wires, etc) which is also available to the person we wish to send a message to. Therefore, it seems that thoughts are formulated in a non-material environment before being translated into a physical environment. This translation, this extra step is rather restrictive. For example, if you imagine a house in your own mind, how many people can see the house? Probably nobody except you. But the house you have imagined is well defined in your mind; you can state its dimensions, its colors, and its materials with stunning precision. By having to represent the house in physical terms (like a drawing, a description, etc), some information is lost. As you imagine the house, its shape can change instantaneously, but once it is translated into a physical environment for others to see, it becomes a rigid structure, i.e. just an instance of all the possible shapes you have thought about. In that sense, the potential of the Universe is not fully utilized at our current level of awareness.
 
Here's a little thought experiment about information and the geometry of thoughts.

Rules
Consider the following mini-game with two players (Blue and Red). On each turn, a player can move one square up, down, left, or right in a 3x3 matrix.
st.png
To win the game, a player must "eat" the other as illustrated below.
ft.png
Thought Experiment
We have already discussed in this thread how the possible combinations/events/states of a game are already stored in the information field (which we are told resides in consciousness), long before we get to mechanistically "compute" them. Discovery seems to be a trademark of the Universe.
Laura said:
(Pierre) Would you say then that the information field contains already ALL possible information?
A: Yes
Click to expand...
Now, let's augment the game by progressively attaching new squares to the top left square of the 3x3 matrix (see below). These newly attached squares are added along a single axis (the y-axis). Obviously, the game will have more possible states as both players will be able to use this new path to attack or escape. It appears as though the initial 3x3 matrix is the starting point from which a straight line is born. But what happens if an infinite number of squares is added? Do the squares end up reconnecting with the starting 3x3 matrix? If you were to tile these squares this way physically (i.e. walk and then place a new square on the ground and so on), they would eventually reconnect with the starting 3x3 matrix because of earth's round shape. Once the circular path is completed, both players are attacking each other and escaping from each other at the same time. There is no more "beginning" and no more "end" to the chase.

dt.png
When we imagine a straight line, is the line necessarily curved in the thought realm?
In other words, is the Universe embedding or translating linear structures into circular structures by default?
Is linearity subjective and circularity objective?
 
An interesting article recently published

Your genetic code has lots of 'words' for the same thing—information theory may help explain the redundancies​

phys.org

Your genetic code has lots of 'words' for the same thing—information theory may help explain the redundancies

Nearly all life, from bacteria to humans, uses the same genetic code. This code acts as a dictionary, translating genes into the amino acids used to build proteins. The universality of the genetic code indicates a common ancestry among all living organisms and the essential role this code plays...
phys.org phys.org

1690885202919.png
 
christx11 said:
Here is my attempt to be more clear. I do not have access to a lot of published sources, so I have tried to use a math paper template for organization and logic, but the post/paper is mainly observational. One of the main intentions of this paper is to basically demonstrate how prime numbers are related to many concepts that are covered in the Cassiopaean sessions transcripts.

It is very difficult to present a theorem and proof to show how a session concept like:

A: What if matter were the "half-life" of energy?

correlates to an observation I have regarding prime numbers.

I hope I have made my observations more understandable in the attached paper/post.

The attachment is a single HTML file in a zip archive.

Attachment:euler_03.zip51,141 bytes
Archived file:euler_03.html204,999 bytes

Unarchive to a temporary directory and open the file with a modern browser like (Edge, Chrome, Firefox, Safari).
Of course scan it, check for malware, whatever you like before opening it.

Older browsers may not work as the HTML file contains the MathJax v3 library.
I would not recommend reading it in a mobile device.

It may take a few seconds to render the first time.

Thanks for your patience.
Click to expand...

I just wanted to update my paper a little. It has been here for over 2 1/2 years with only two responses. One in this thread and one other response in the April 23rd 2022 session thread. Ark kind of originally requested this paper and up until a few months ago I thought Ark had read it even though I received no response from him. The two responses I did receive just seemed weird.

Anyway I decided a couple of months ago to try and see if I could get a greater audience to perhaps take a look at the paper and I revived an old Blog site with the story of this paper. The version I am going to present in the Blog has several typos fixed and some context switching fixed and a few other small changes. I wanted to upload that updated version here also so that they were in sync. Eventually I have some contacts via work and family to universities that I will try to get some other eyes on it and hopefully someone can answer my questions.

If you are interested in the background of this paper and its journey here on this forum you can check it here.

TimeFree

As far as the new version I am uploading here:

There are not many changes.
Fixed typos,
Fixed context switching through Section 2,
Added a couple of additional excerpts,
Removed a small piece I did not think necessary.

The attachment is a single HTML file in a zip archive again.

Zip Archive Compressed Size
Attachment:primes.zip3,822,682 bytes

The zip file is much larger then before, as it carries the Mathjax JavaScript library and image files, so that the html file can be viewed offline.

Download the zip archive. Scan it. Unzip it to a temp directory like c:\temp.
Open Windows Explorer, go to C:\temp\primes and double click on the file euler_03.html.
 

Attachments

Have started reading, and it is not easy for me. Can you help me by first telling me if you have any problem with the proof of the Euler product formula, as it is done, for instance, in this document:


The formula (1) in your paper is not the original Euler product. It is an expression that does not have any obvious meaning. Looks like an attempt to divide infinity by infinity, and there are probably infinitely many ways to try to give it a well defined meaning. So, please help me to understand your intentions.
 
Last edited:
ark said:
Have started reading, and it is not easy for me. Can you help me by first telling me if you have any problem with the proof of the Euler product formula, as it is done, for instance, in this document:


The formula (1) in your paper is not the original Euler product. It is an expression that does not have any obvious meaning. Looks like an attempt to divide infinity by infinity, and there are probably infinitely many ways to try to give it a well defined meaning. So, please help me to understand your intentions.
Click to expand...

I appreciate you taking the time to help me. I will try to make myself clear and hope that I can do so. Talking to me about this paper of mine is probably going to require you to imagine you talking to yourself when you were 14 or 15 years old. I know the so called math paper I have produced is going to be difficult to follow. I will try to explain.

First formula 1.


eq_01.png

I used label 1 more for a clickable link for reference later on.

In the introduction I started with a proper equality, the Euler conclusion.

euler_product_01.png

Euler began with the harmonic series ( left side of the equation ) and his result was the infinite product on the right side of the equation. Euler showed that the harmonic series was equal to this infinite product on the right hand side.

At section 2, of my paper I am just trying to say, I want to reverse this process that Euler used. I want to start with Euler's infinite product, his result/conclusion and try and see if I can recover the harmonic series from the infinite product. Label (1) is just saying let the infinite product be our starting point and let's see if we can recover the harmonic series from Euler's infinite product.

I hope that makes sense.

In regards to the paper you have provided the link to. Yes, I can pretty much follow it fine. But to get to my main point of the my paper we can probably restrict my paper to needing to read only through section 2. All sections following are built from the logic in section 2 and if that logic fails, then all the following sections fail. If I can make my point here up through section 2, then and only then do we even need to proceed further.

To make my point, which is actually very simple, in the paper you have linked to, we do not need to go any further than the first few sentences of section 1.1.

euler_product_02.png

The crux of my entire argument/confusion does not need to go any further than the above portion of the linked paper.

I need to add more to this explanation and I will, but I have only small windows of time during my work hours for my job to make responses so it may take a few days and several small posts to try to explain myself.

As regards my paper, you do not have to read any further than section 2 and all of what I have in section 2 fits with the part of the paper you linked to and only the section (very beginning of the paper) that I posted in the above image.

I will try to explain that in the next post and maybe my confusion can be put to bed rather quickly.

Thanks
 
My next post. I am trying to figure out a way to make my posts easy to refer back to and in the forum environment that is challenging.
In this post I am just going to post an image of the linked pdf document Ark posted, the first few lines that are pertinent to my paper and questions / confusion. Hopefully then I can refer back to this post with a post number link and it will be easier to refer back to it for reference by clicking on the post link and then returning to the post the link came from by hitting the back history button or back arrow in the browser to return to the originating post.

Here is the image once again of the paper Ark linked to with 3 specific lines pointed to that I will make reference to.

euler_product_03.png
 
To continue trying to explain my paper. I left off answering what is formula (1)?
Here at the beginning of section 2, I am just trying to say I want to start with Euler's infinite product (the result that he derived from the infinite summation series of inverse naturals / the harmonic series) and I want to attempt to prove to myself that I can reverse the process and start with the infinite product result and recover the harmonic series (what Euler started with).

In section 2.1 I am just trying to state what condition(s) must exist to falsify this reverse process.

2.1 Falsification criterion 1, is simply saying that if I cannot recover/re-create all elements of the inverse naturals / harmonic series, then Euler's theorem 7 cannot be true.

2.2 Falsification criterion 2, simply says that I can recover all elements of the inverse naturals / harmonic series, but in addition to that, Euler's infinite product also creates at least one number that is not a member of the harmonic series / all inverse natural numbers, then Euler's theorem 7 is false.

If either of these conditions are true, then Euler's theorem 7 is false.

In simple terms I am saying that if I reverse a process I should be able to get back exactly what I started with. If I get back less than what I started with, then something is wrong. Also if I get back more than I started with, then something is wrong.

2.3 The Euler product is a product of prime power series.

Here in section 2.3 I am trying to prove to my 14-15 year old self that Euler's infinite product result can be written as an infinite product where each term of the infinite product is an inverse prime power series. I am very visually oriented and I am 14 years old and I want to go through the logic that tells me, yes, Euler's infinite product can be represented as an infinite product of inverse prime geometric power series.

I end up demonstrating to myself that yes the Euler product can be represented as an infinite product where each term is an inverse prime geometric power series. That result is identified by label (2). Label (2) is the same thing as Label (1), it is just Euler's infinite product written as an infinite product of inverse prime geometric power series.

All of that, getting from Label (1) to Label (2) is simply expressed in the external linked document (Post #446 ) by balloon callout (3).

euler_product_04.png

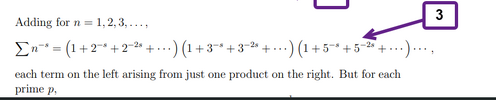
The equation / formula in balloon callout (3) on the left side is the infinite summation series of inverse naturals numbers (the harmonic series) and it is equal to on the right hand side an infinite product of inverse prime power series.

So in my 14 year old mind I have shown myself that callout (3) in the external reference is indeed true. In my paper I went From Label (1) to Label (2) to show that the harmonic series = Euler's infinite product = an infinite product of inverse prime geometric power series.

The only difference between the result in the external reference callout (3) equation right side infinite product of inverse prime power series and my representation in Label (2) of my paper, is that the external reference writes each term of the infinite product of inverse prime power series horizontally and I write each term of the infinite product of prime power series vertically.

The right side of external callout (3) written in my paper at Label (2):

euler_product_05.png
I prefer this vertical representation visually of Euler's product because it makes it easier visually for me to see the models I want to use for trying to recover all term/elements of the harmonic series. I want to use the above visual representation as one method by tracing distinct / unique multiplicative distributive paths that align with the second method, infinite multiplicative sequences.

The values for for each term of an infinite multiplicative sequence lines up with each term or column per se of the above infinite product of prime geometric power series. It makes it easier to see each representation.

Here I will once again refer to the external reference document which I have in Post #446, and balloon callouts (1) and (2).
callout_2_3.png


Callout (1) is the infinite canonical form of factorization of any natural number (positive integer).
Callout (2) is the infinite canonical form of factorization of any inverse natural number (inverse positive integer).

I have one issue with the terminology above and that is with the phrase in paratheses (all but a finite number being 0). To me that statement is difficult to understand. It is saying, all exponents being equal to zero, except for a finite number of terms not being 0. I would say it as (with a finite number of exponents being non-zero), that means in the infinite sequence you can only have a finite number of the infinite terms raised to power/exponent that are not a value of 0, as that is the actual the definition of a natural number. A natural number is finite. If it had infinite terms in its canonical factorization form where the exponent was not 0, then the number would not be finite, it would be an infinite number, not a natural number.

The infinite multiplicative sequence or infinite product representation of every natural number (positive integer) tells us that every natural number represented in this way has what I will call a signature. To represent every natural number as an infinite product (By the Fundamental Theorem Arithmetic) tells us that in that infinite rightward progression of terms (the next prime raised to a power of either 0 or a positive integer value), that somewhere in that infinite progression to the right, all exponent values must be 0. If you think of the largest positive integer you can think of and you factor it in its canonical infinite form, it will have some largest prime number raised to a power/exponent that is not a 0, but after that every prime in the infinite product will have an exponent of 0. This is the signature of every natural number (positive integer). The Fundamental Theorem of Arithmetic requires it by definition.

In the externally linked document, callout (2), all the same rules apply for the infinite canonical representation of factors in the inverse natural numbers. The only difference is, for the exponents we are using 0 or a negative integer value rather than a positive integer value. So we have a similar type of signature available in the inverse natural numbers.

Back to my paper, we are basically at Remark 3. In Remark 3, I am once again talking to my 14 year old self trying to understand the Fundamental Theorem of Arithmetic and this idea that every natural number or inverse natural can be represented as an infinite multiplicative sequence or infinite product in this infinite canonical factorization form and it leads to a contradiction that I see and that I am trying to express in sections 2.4 and 2.5.

In my next post I will try to express that contradiction and it really comes to the point of my entire paper, of what I am trying to understand, and why or why not what I see is true.
 
That doesn't help me at all. Words, words, words. I am getting lost. Try to skip all that is not absolutely essential. So, please, remove from your paper all introductions, side remarks, unnecessary formulas, words. Leave what is essential and can not be removed. Just pure math. Then it will be easier to discuss the problem. Otherwise we will get lost in a forest of words. And then we can discuss it, sentence by sentence, one sentence at a time. And if you have questions, ask, again one question at a time.
 
Last edited:
ark said:
That doesn't help me at all. Words, words, words. I am getting lost. Try to skip all that is not absolutely essential. So, please, remove from your paper all introductions, side remarks, unnecessary formulas, words. Leave what is essential and can not be removed. Just pure math. Then it will be easier to discuss the problem. Otherwise we will get lost in a forest of words. And then we can discuss it, sentence by sentence, one sentence at a time. And if you have questions, ask, again one question at a time.
Click to expand...
The crux of the matter.

Here is an illustration of Euler's infinite product is its expanded prime power series form.
By the fundamental Theorem of Arithmetic I can see that it contains all the elements of the harmonic series, every element of the inverse naturals.
finite_paths.png


The below illustration is the same as the illustration above, but it contains elements/numbers that are creatable that first principles cannot account for.

infinite_paths.png
Here in 2.5, every number is infinite.
Convince me that they do not exist.

There is nothing wrong with the first principles, they do everything they say and are defined to do. But they do not account for these infinitely factored numbers or infinite numbers. I would like to know why I should ignore them and pretend they do not exist.
 
You must log in or register to reply here.

Trending content

Back
Top Bottom